Just intonation: building intervals

The just intonation series is back! Before I start, I have a quick observation that I forgot to mention in the last post on the topic. So far, we have only looked at a single interval, the octave, derived from the ratio 2:1. So let’s continue up the harmonic series and see what else we can find.

Since we left off with a factor of two, the next ratio in the series would be a factor of three, or 3:1. Applying this ratio to a 100 Hz frequency would give us 300 Hz. These two pitches give us the interval of an fourteenth, that is, an octave plus a perfect fifth. If we now divide by our 2:1 ratio to move the pitch down by an octave, we end up with another ratio we can use, 3:2, which is our familiar perfect fifth.

For 4:1, we end up with an interval of two octaves. This makes sense if we consider that 4 = 2 × 2, which means 4:1 is just two 2:1 intervals (octaves) stacked together. This is the start of a pattern in the harmonic series. Any time a ratio in the series can be made by multiplying earlier ratios together, its resulting interval will be the same as stacking the intervals of the earlier ratios. This is due to the equivalence between the multiplication of ratios and the addition of traditional intervals, as discussed in the first article.

Let’s go one more step up the harmonic series before bringing it all together. A 5:1 ratio cannot be produced by multiplying previous ratios together, so that means whatever a factor of 5 produces, it can’t be created just by stacking octaves and perfect fifths. The result of a 5:1 ratio is two octaves plus a major third. If we divide by four to remove the octaves, like we did with 3:2 above, we end up with the major third, with a ratio of 5:4.

Here’s a chart summing up all the ratios we’ve looked at so far and beyond, up to 10:1. I note the ratio, the factorization (if applicable), and the musical interval associated with each step of the series. For simplicity’s sake, I am taking advantage of octave equivalency and regarding intervals that only differ by octaves as equivalent. For example, 3:1 and 6:1 are both marked as perfect fifths, because either one can be a perfect fifth (3:2) if you divide by two enough (6:1 / 2 / 2 = 6:4 = 3:2).

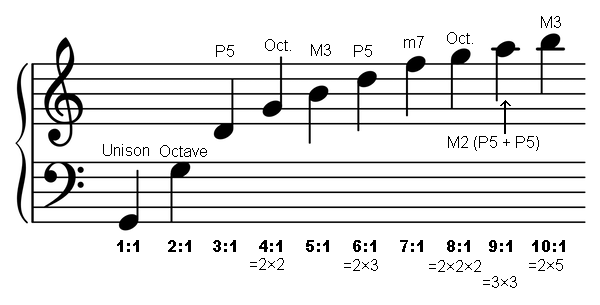
The important takeaway I want to leave you with is this: intervals based on composite numbers can be built from existing intervals (for example, 9:1 shows how you can get a major second by stacking perfect fifths), but whenever you have a prime-numbered ratio, it introduces a new type of interval that hasn’t been seen in the series up to that point. For primes in this chart, we have 2:1 introducing the octave, 3:1 introducing the perfect fifth, 5:1 introducing the major third, and 7:1 introducing the minor seventh.

I consider this to be a key insight in navigating the world of just intonation confidently, but we’ll have to wait until next time to really unpack that insight. I’d also like to explore how we can derive all the other intervals we might need just with what we already have in front of us here.

Have you ever tried to explain something you thought was really interesting but it turned out to be more complicated than you realized once you opened your mouth and you wondered if you were even making any sense? Let me know your thoughts at my Ctrl-C email: gome ​@ ​ctrl-c.club.

Next post in series